- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
CIE A Level Maths: Pure 3复习笔记8.1.4 Complex Roots of Polynomials
Complex Roots of Quadratics
What are complex roots?
- Complex numbers provide solutions for quadratic equations which have no real roots
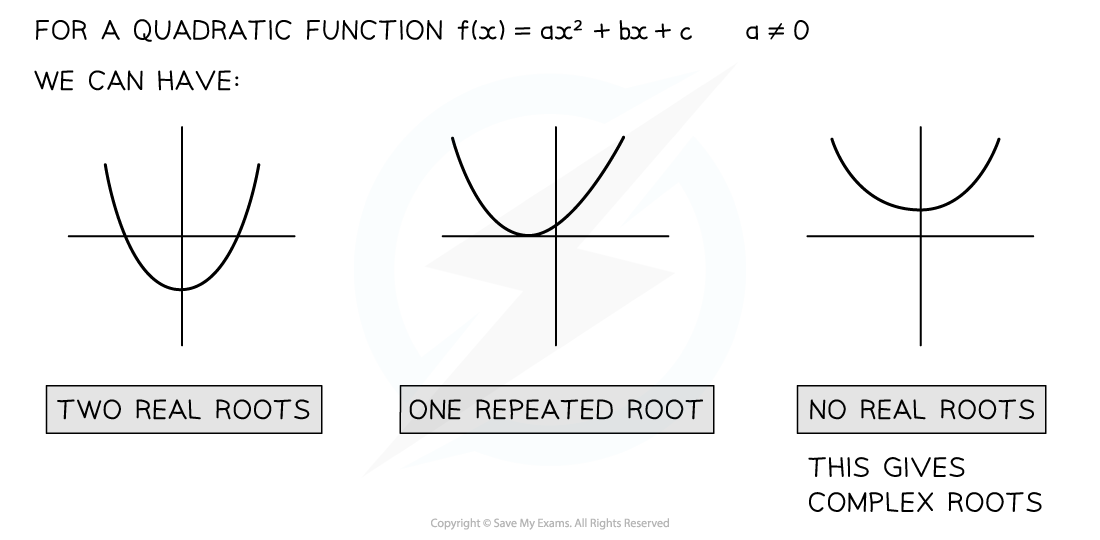
- Complex roots occur when solving a quadratic with a negative discriminant
- This leads to square rooting a negative number
How do we solve a quadratic equation with complex roots?
- We solve an equation with complex roots in the same way we solve any other quadratic equations
- If in the form
 we can rearrange to solve
we can rearrange to solve - If in the form
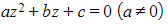 we can complete the square or use the quadratic formula
we can complete the square or use the quadratic formula
- If in the form
- We use the property
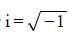 along with a manipulation of surds
along with a manipulation of surds
- When the coefficients of the quadratic equation are real, complex roots occur in complex conjugate pairs
- If
 is a root of a quadratic with real coefficients then
is a root of a quadratic with real coefficients then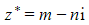 is also a root
is also a root
- If
- When the coefficients of the quadratic equation are non-real, the solutions will not be complex conjugates
- To solve these use the quadratic formula
How do we find a quadratic equation given a complex root?
- We can find the equation of the form
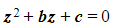 if you are given a complex root in the form
if you are given a complex root in the form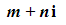
- We know that the complex conjugate
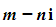 is another root,
is another root, - This means that
 and
and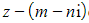 are factors of the quadratic equation
are factors of the quadratic equation - Therefore

- Writing this as
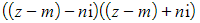 will speed up expanding
will speed up expanding
- Writing this as
- Expanding and simplifying gives us a quadratic equation where b and c are real numbers
- We know that the complex conjugate
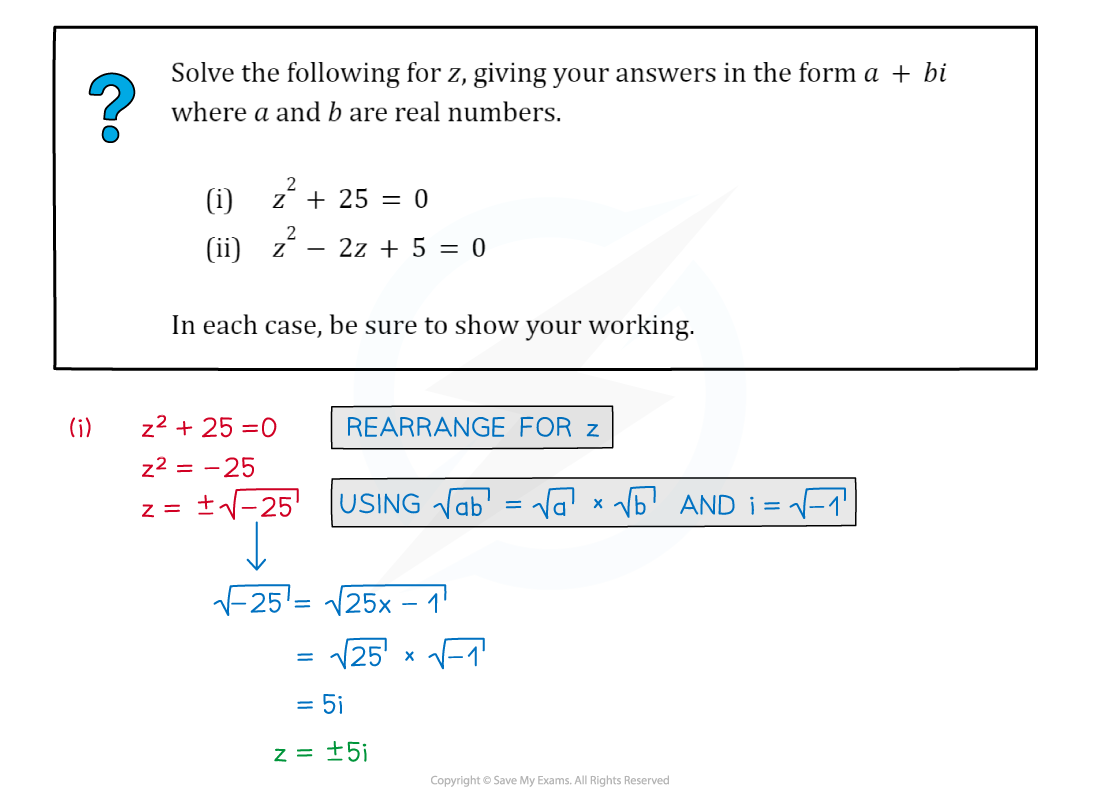
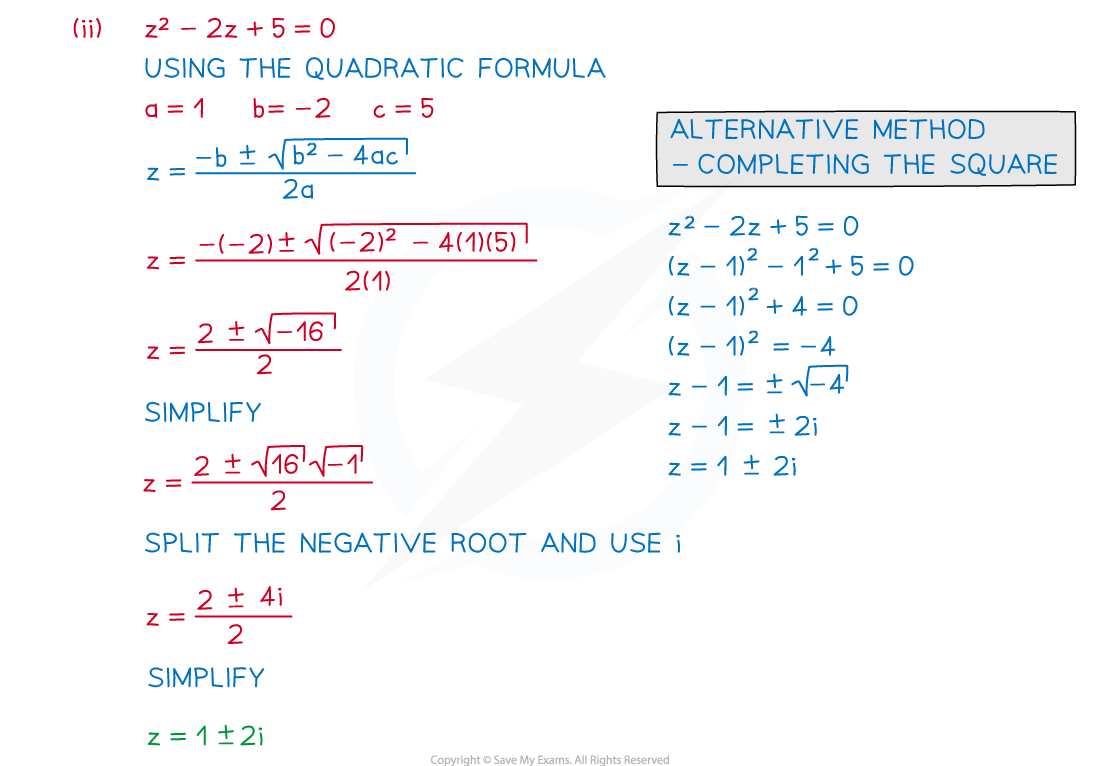
Worked Example
Exam Tip
- Once you have your final answers you can check your roots are correct by substituting your solutions back into the original equation.
- You should get 0 if correct! [Note: 0 is equivalent to 0 + 0i]
Complex Roots of Cubics & Quartics
How many roots should a polynomial have?

How do we solve a cubic equation with complex roots?
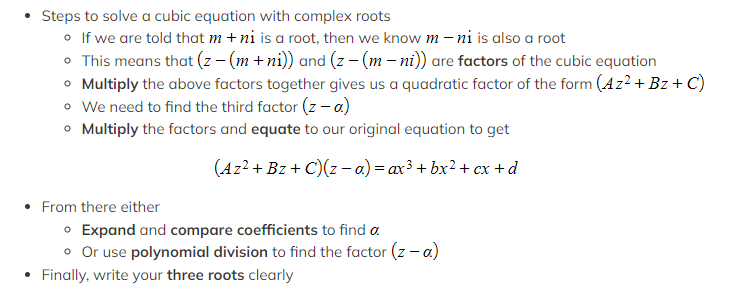
How do we solve a quartic equation with complex roots?

How do we solve cubic/quartic equations with unknown coefficients?

Worked Example
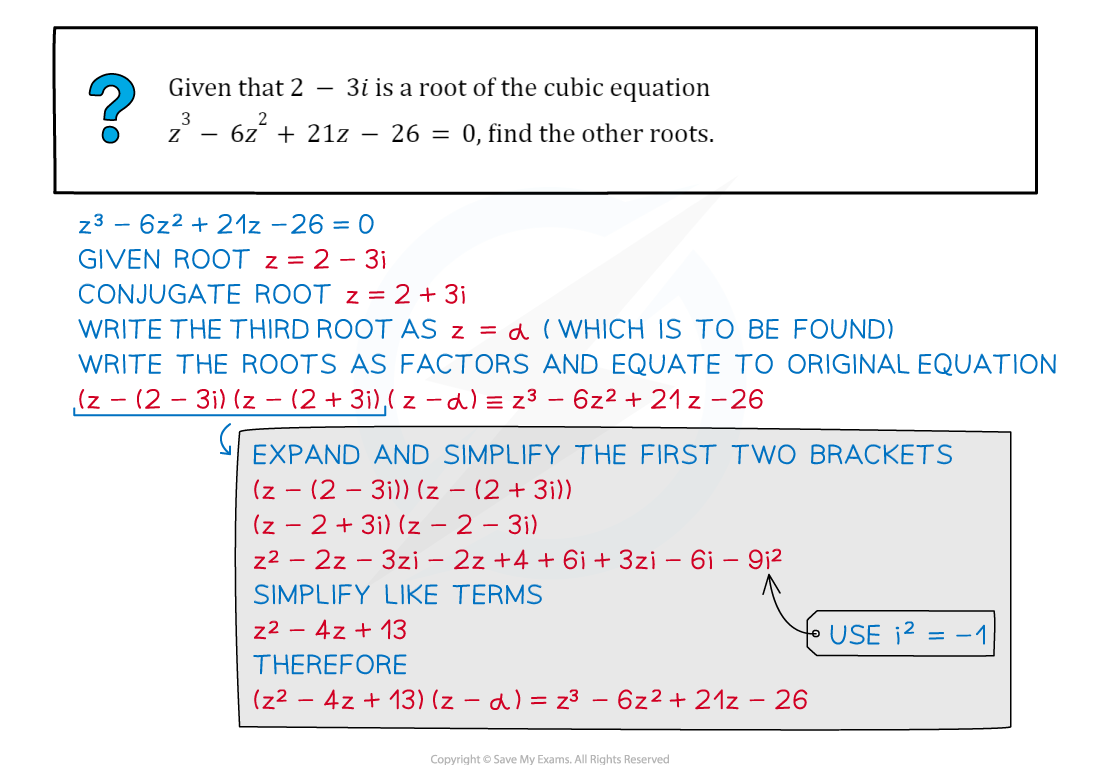
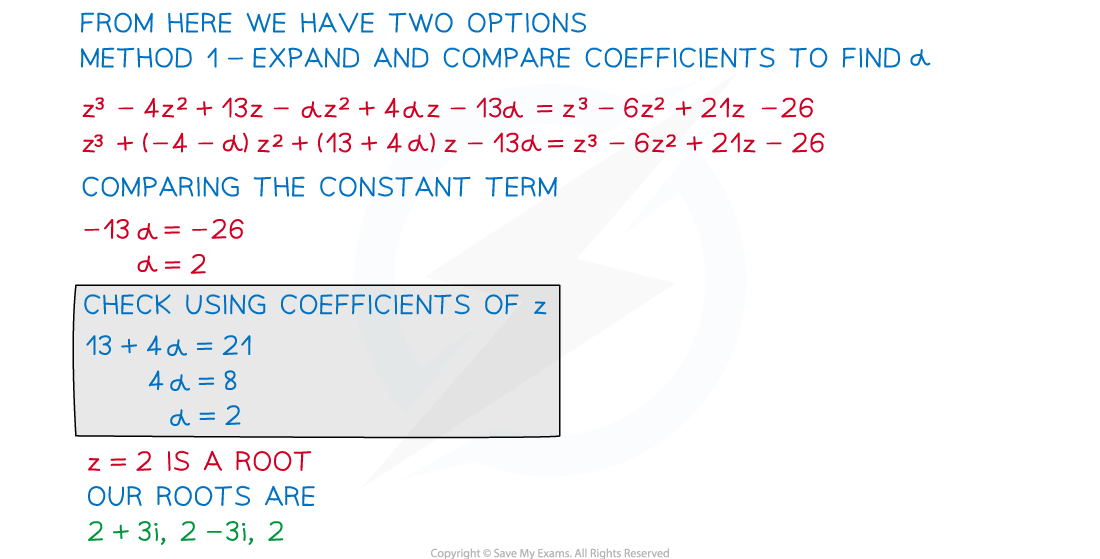
转载自savemyexams
站内搜索
竞赛真题免费下载(点击下载)
在线登记
最新发布
© 2024. All Rights Reserved. 沪ICP备2023009024号-1





