- 翰林提供学术活动、国际课程、科研项目一站式留学背景提升服务!
- 400 888 0080
IB DP Physics: SL复习笔记4.2.4 Sound Waves
Sound Waves
- Sound waves are longitudinal waves and, as such, require a medium in which to propagate
- Sound waves are generated by oscillating sources, which produce a change in density of the surrounding medium
- The sound wave then travels with a series of compressions and rarefactions
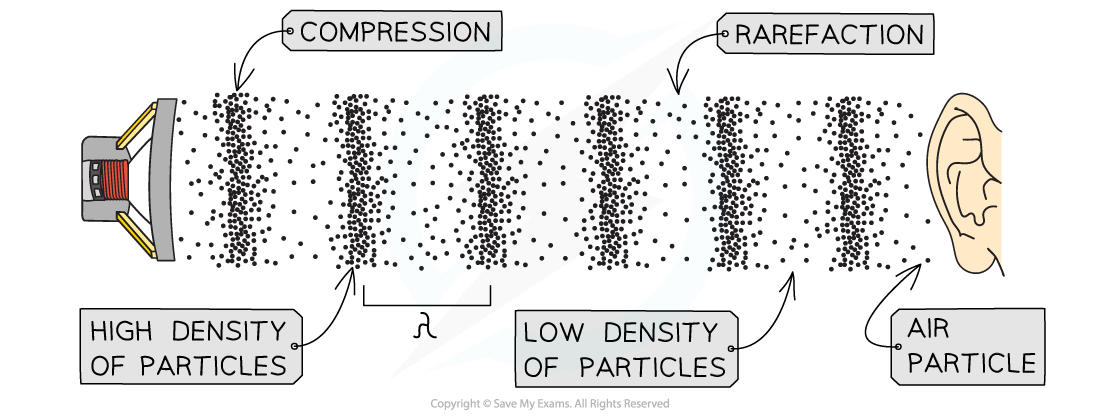
A sound wave travelling through air
- Sound waves form a continuous spectrum based on their frequency
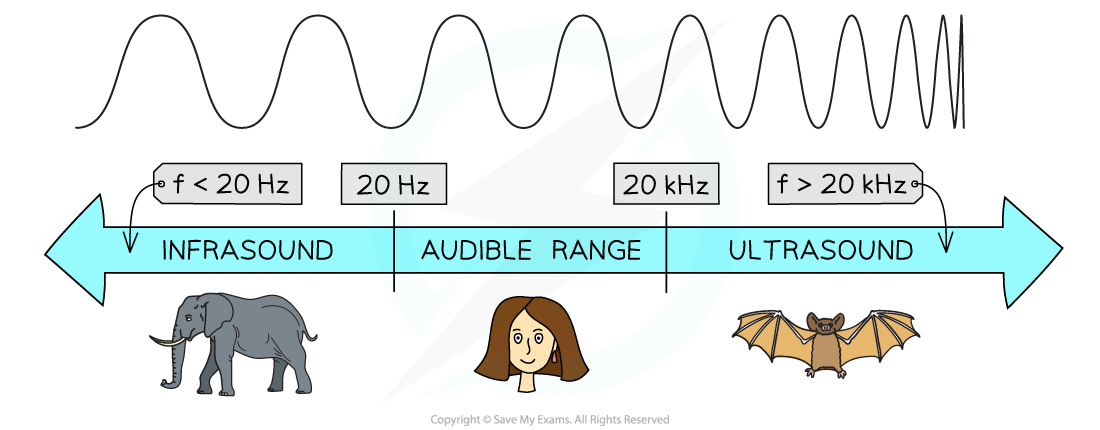
The spectrum of sound waves
- Humans can only hear sounds with frequencies in the range 20 Hz - 20 kHz, known as the audible range
- Sounds with frequencies below and above this range cannot be detected by the human ear
Pitch and Volume
- The frequency of a sound wave is related to its pitch
- Sounds with a high pitch have a high frequency (or short wavelength)
- Sounds with a low pitch have a low frequency (or long wavelength)
- The amplitude of a sound wave is related to its volume
- Sounds with a large amplitude have a high volume
- Sounds with a small amplitude have a low volume
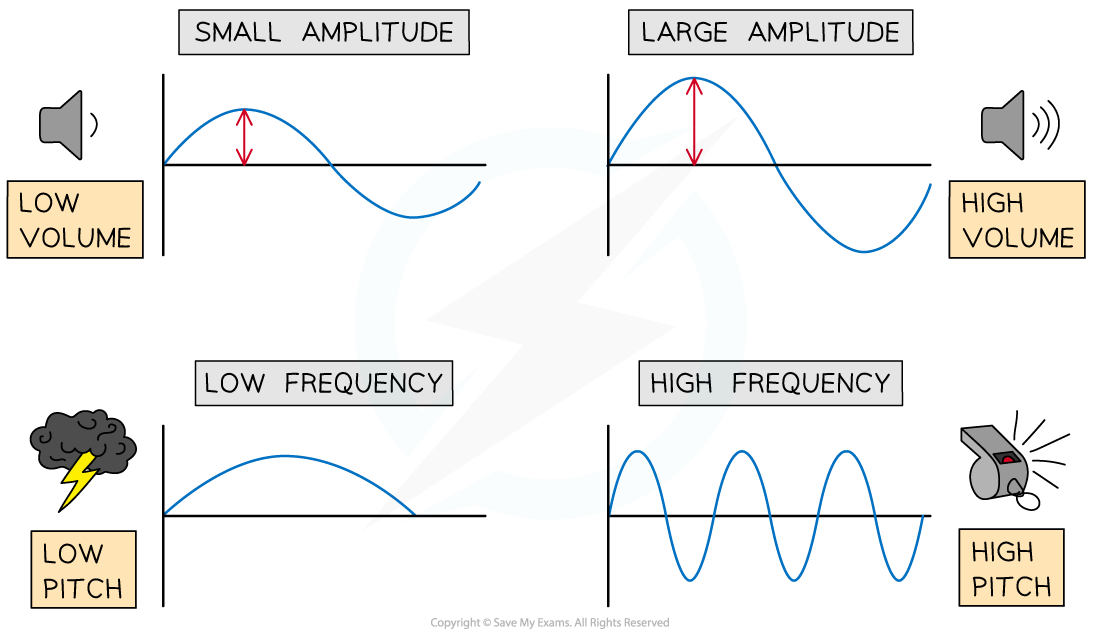
Pitch and amplitude of sound
Speed of Sound
- Sound waves travel at a speed of about 340 m s–1 in air at room temperature
- The higher the air temperature, the greater the speed of sound
- The is because the average kinetic energy of the particles is higher
- Sound travels the fastest through solids, since solid particles are closely packed and can pass the oscillations onto their neighbours much faster
- Sound travels the slowest in gases, since gas particles are spread out and less efficient in transferring the oscillations to their neighbours
Echo
- Sound waves reflect off hard surfaces
- This phenomenon is known as echo
- Echo can be used to obtain an experimental value of the speed of sound. This is calculated using the equation
![]()
- Where:
- v = speed of sound in metres per second (m s–1)
- d = distance between the sound source and the hard surface (m)
- t = time taken to travel from the source to the hard surface and back (s)
Measuring the Speed of Sound Experimentally - Fast Timer
- The speed of sound can be measured using a fast timer (one which can measure to the nearest millisecond or even microsecond)
- Two microphones separated 1 m apart are connected to a fast timer
- The first microphone triggers the timer to start
- The second microphone triggers the timer to stop
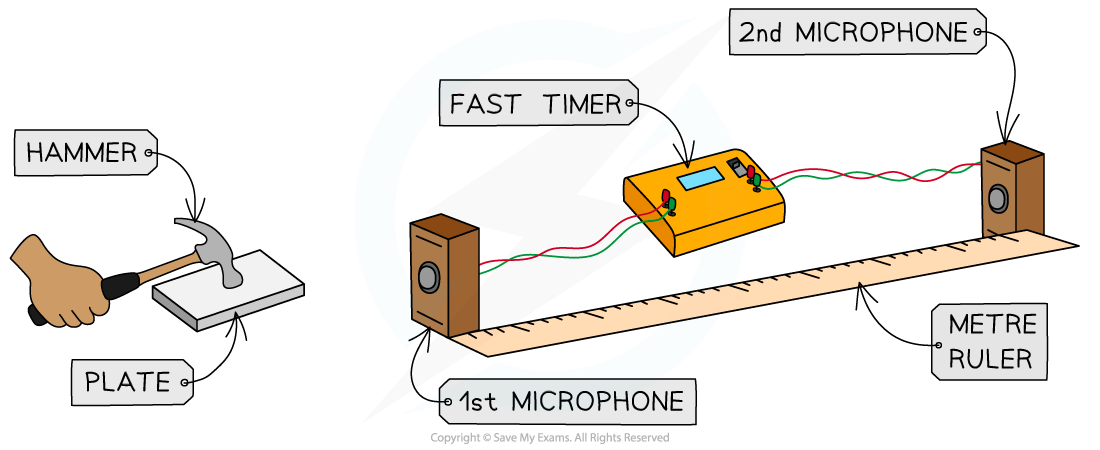
- A hammer is made to strike a plate
- The sound waves from the plate travel to the two microphones triggering the first and then the second
- The time delay will be around 3.2 ms
- The speed of the waves can be calculated by rearranging the equation: distance = speed × time
Measuring the Speed of Sound Experimentally - Double Beam Oscilloscope
- Two microphones are connected to the input of a double beam oscilloscope
- A signal generator is connected to a loudspeaker and set to a frequency between 500 Hz and 2.0 kHz
- One of the microphones is close to the loud speaker
- The other microphone is 1 m away

- There will be two traces that appear on the screen
- The traces are compared as the second microphone is moved back and forth in line with the first microphone and the speaker
- Use a ruler to measure the distance that the second microphone needs to move for the traces to be in phase then out and phase and back in phase again
- This distance is equal to the wavelength of the wave
- The speed of the waves are therefore calculated using c = fλ
Worked Example
A person stands 50 m from a wall. The person claps their hands repeatedly, and changes the clapping frequency until the echoes are synchronised with the claps. A mobile phone application measures the time between the claps, which is t = 0.30 s. Determine the speed of sound.
Step 1: Write down the known quantities
-
- Distance between the person and the wall, d = 50 m
- Time between the claps, t = 0.30 s
Step 2: Write down the "echo equation"
![]()
Step 3: Substitute the numbers into the above equation and calculate the speed of sound v
![]()
v = 330 m s–1
转载自savemyexams
站内搜索
竞赛真题免费下载(点击下载)
在线登记
最新发布
© 2024. All Rights Reserved. 沪ICP备2023009024号-1





