Remind me of the rule for differentiating powers

• If y = kxⁿ then dy/dx = knx^n-1

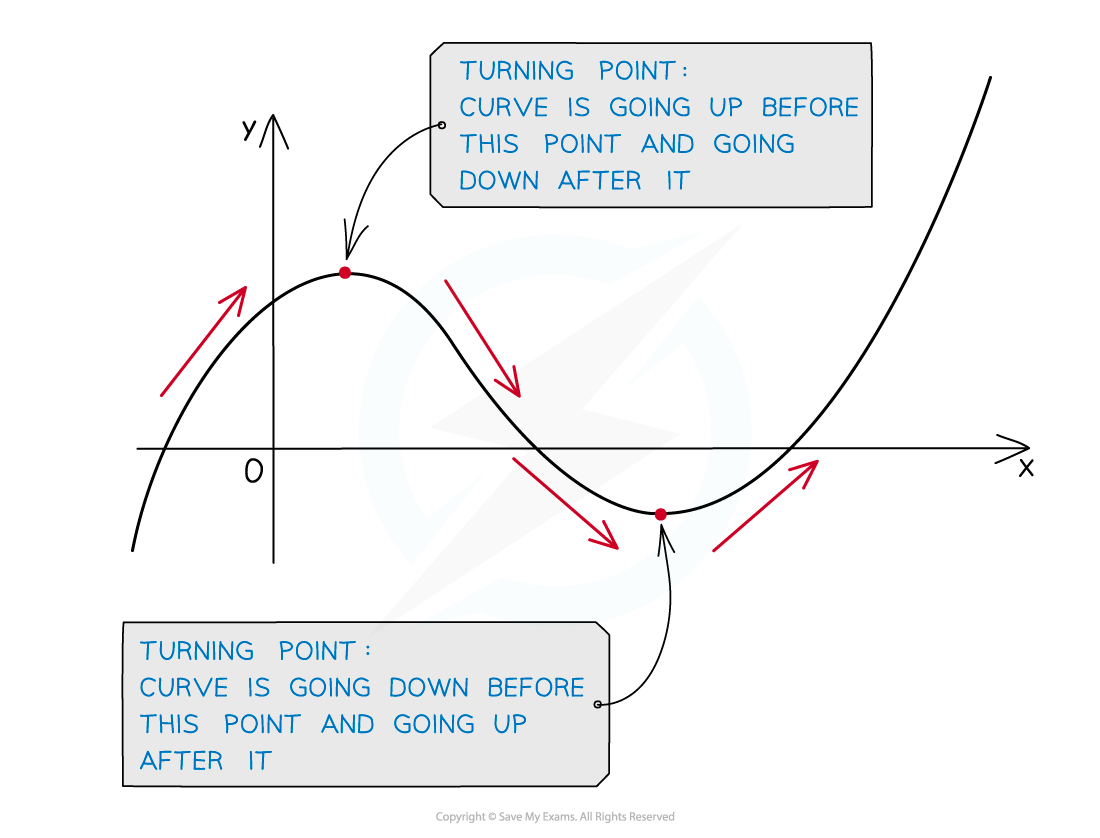
What is a turning point?

• The easiest way to think of a turning point is that it is a point at which a curve changes from moving upwards to moving downwards, or vice versa
• Turning points are also called stationary points
• Ensure you are familiar with Differentiation – Basics before moving on

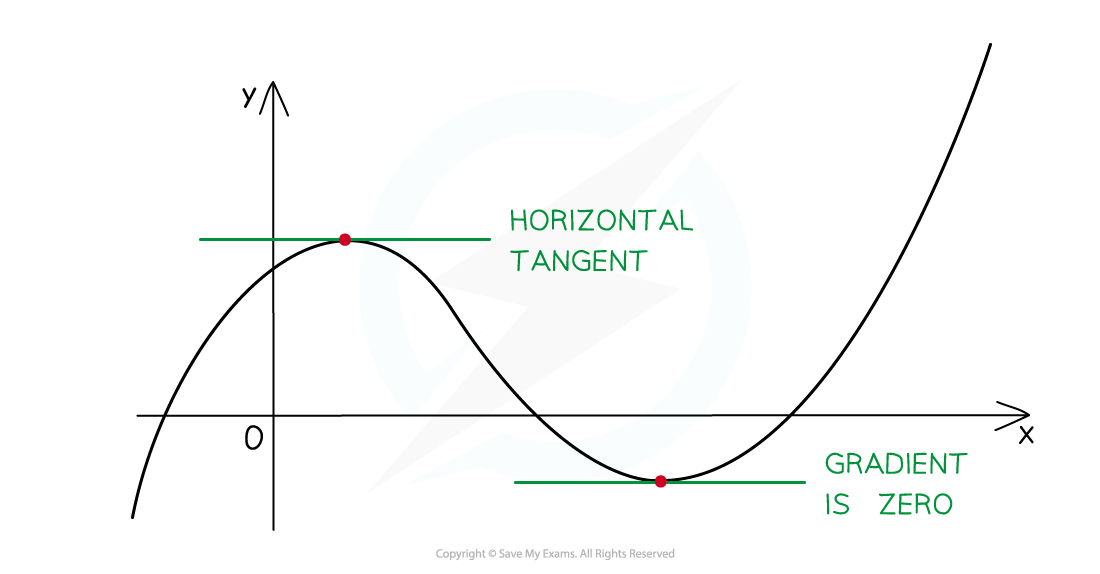
• At a turning point the gradient of the curve is zero
  • If a tangent is drawn at a turning point it will be a horizontal line
  • Horizontal lines have a gradient of zero

   

• This means at a turning point the derivative or gradient function equals zero

How do I know if a curve has turning points?

• You can see from the shape of a curve whether it has turning points or not
• At IGCSE, two types of turning point are considered:
  • Maximum points – this is where the graph reaches a “peak”
  • Minimum points – this is where the graph reaches a “trough”

   

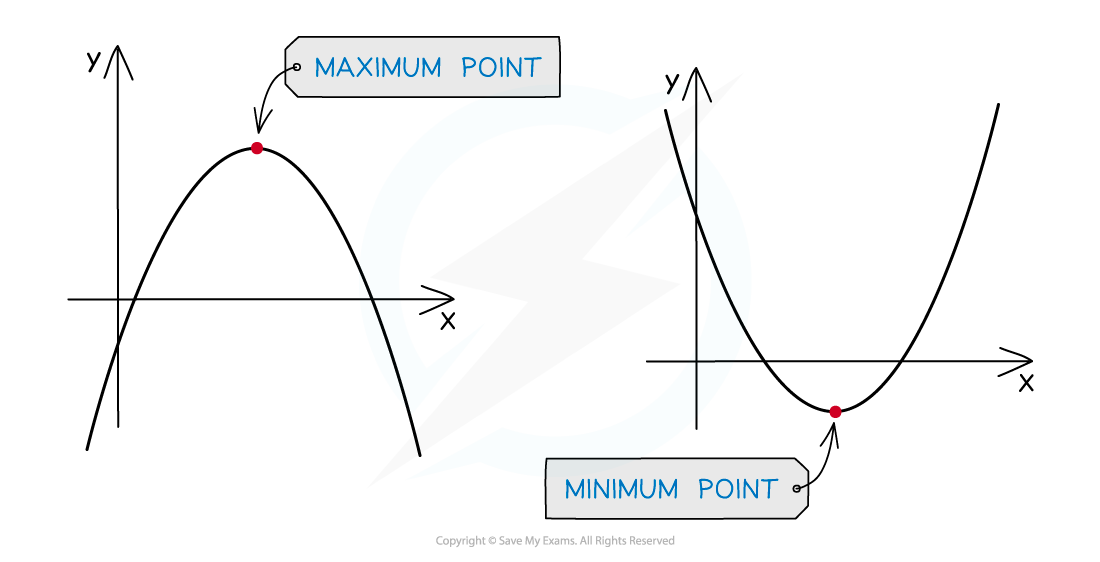
• These are sometimes called local maximum/minimum points as other parts of the graph may still reach higher/lower values

How do I find the coordinates of a turning point?

• STEP 1  Solve the equation of the gradient function (derivative) equal to zeroie. solve dy/dx = 0

  This will find the x-coordinate of the turning point

• STEP 2  To find the y-coordinate substitute the x-coordinate into the equation of the graph 

  ie. substitute x into “y = ...”

How do I know which point is a maximum and which is a minimum?

• The easiest way to do this is to recognise the shape of the curve
  • ... either from a given sketch of the curve
  • ... a sketch of the curve you can quickly draw yourself 

    (You may even be asked to do this as part of a question)

  • ... the equation of the curve

   

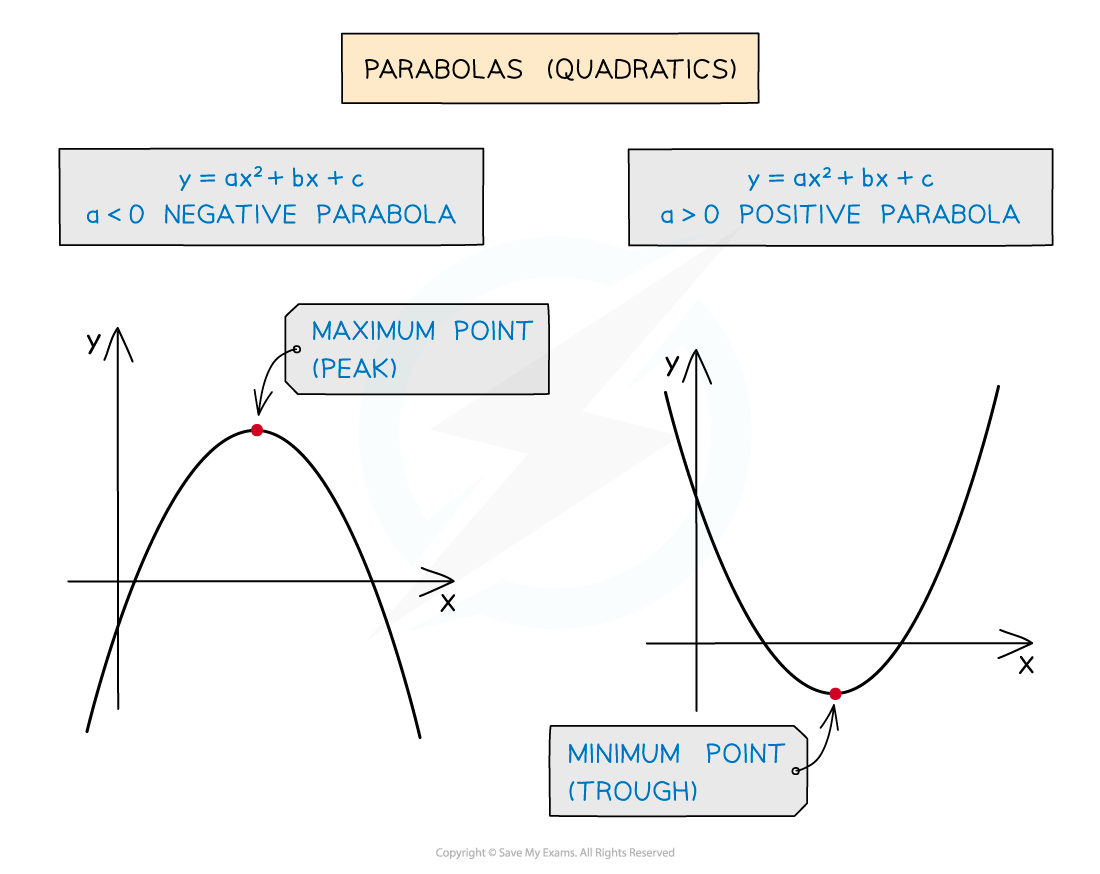
• For parabolas (quadratics) it should be obvious ...
  • ... a positive parabola (positive x² term) has a minimum point
  • ... a negative parabola (negative x² term) has a maximum point

   

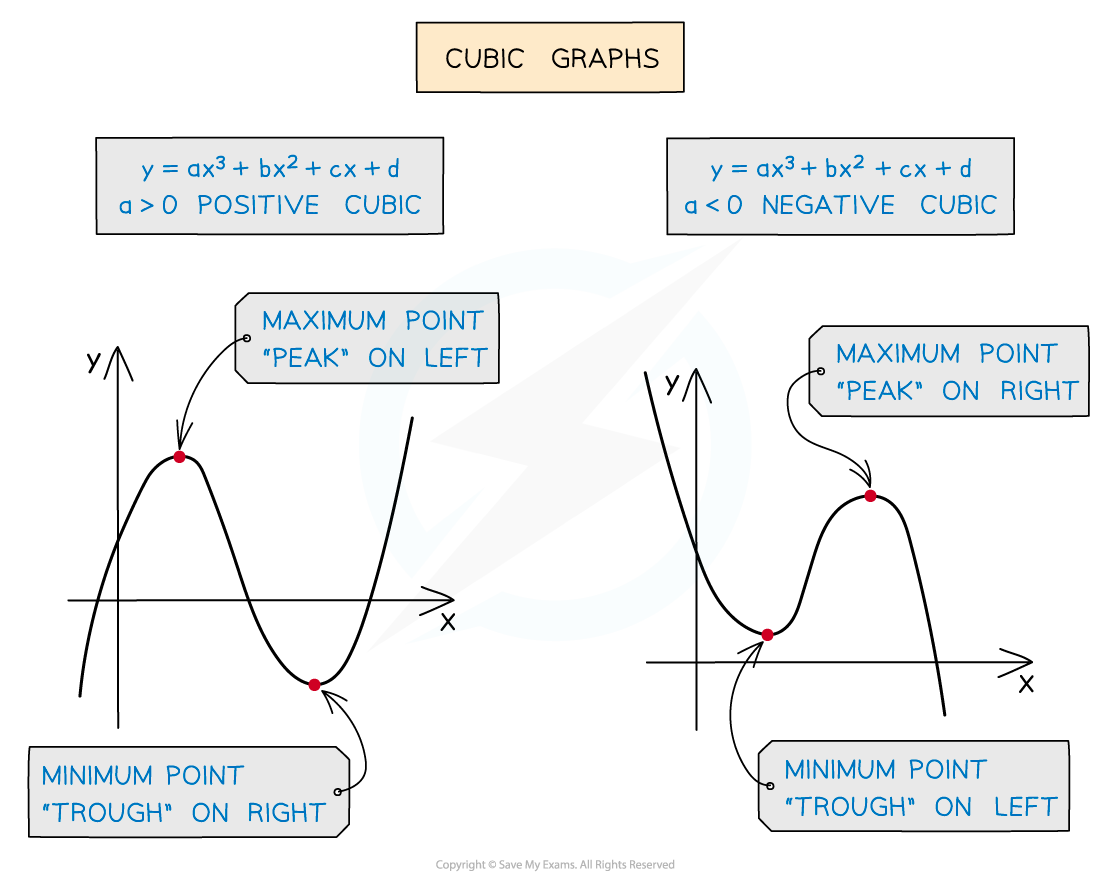
• Cubic graphs are also easily recognisable ...
  • ... a positive cubic has a maximum point on the left, minimum on the right
  • ... a negative cubic has a minimum on the left, maximum on the right