Edexcel IGCSE Maths 复习笔记 2.15.1 Quadratic Simultaneous Equations
What are quadratic simultaneous equations?
- When there are two unknowns (say x and y) in a problem, we need two equations to be able to find them both: these are called Simultaneous Equations
- If there is an x2 or y2 in one of the equations then they are Quadratic (or Non-Linear) Simultaneous Equations
(They can be represented by a straight line and a curve on a graph – the two pairs of answers are the points of intersection of the line and the curve)
How do you solve quadratic simultaneous equations?
- This is called the Substitution Method:Label the equations A and B
- Rearrange the linear equation to y = … (or x = …)
- Substitute for y (or x) in the quadratic equation
- Multiply out brackets
- Rearrange to “quadratic = 0”
- Solve using appropriate method (Factorisation or Formula)
- Substitute back into the linear equation to find the other variable
- Check your answer by substituting into the equation you didn’t use in 6
Exam Tip
If the resulting quadratic has a repeated root then the line is a tangent to the curve.If the resulting quadratic has no roots then the line does not intersect with the curve – or you have made a mistake!When giving your final answer, make sure you indicate which x and y values go together. If you don’t make this clear you can lose marks for an otherwise correct answer.
Worked Example
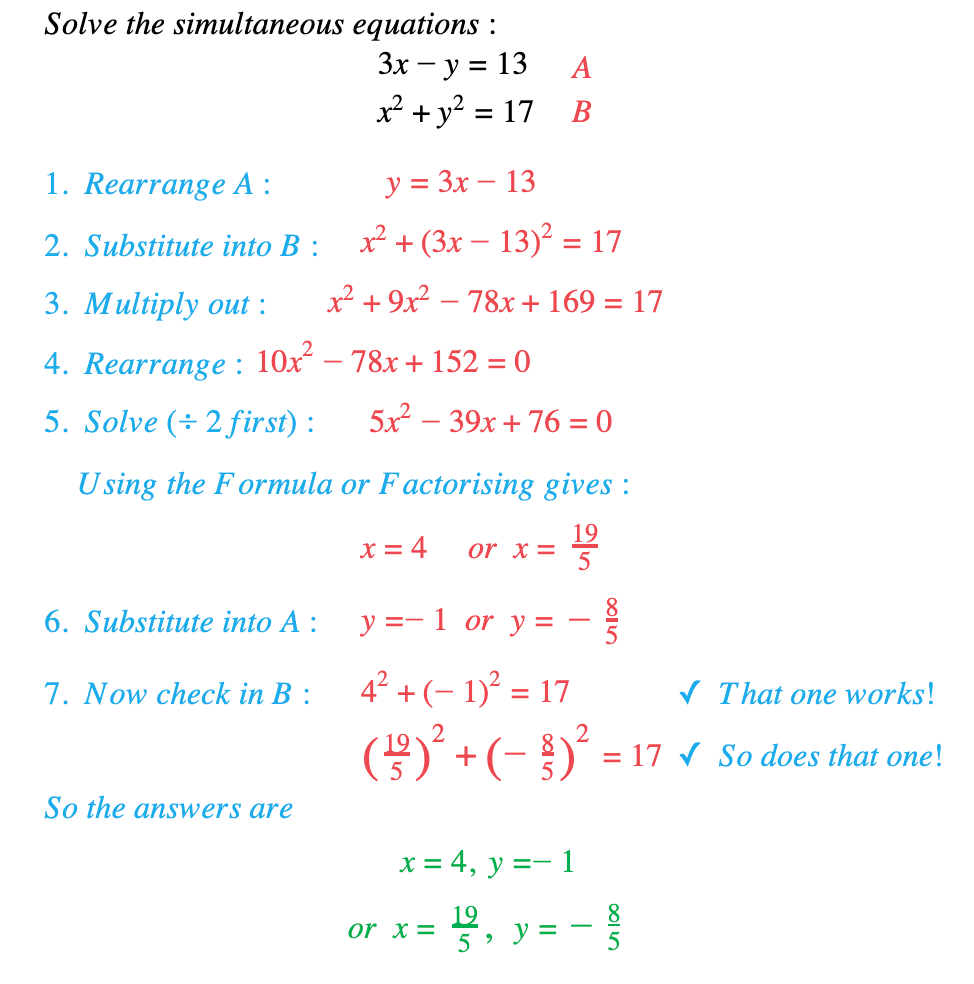
转载自savemyexam
